106. Construct Binary Tree from Inorder and Postorder Traversal

Posted on Edited on In

106. Construct Binary Tree from Inorder and Postorder Traversal

Description

Difficulty: Medium

Related Topics: Array, Hash Table, Divide and Conquer, Tree, Binary Tree

Given two integer arrays inorder and postorder where inorder is the inorder traversal of a binary tree and postorder is the postorder traversal of the same tree, construct and return the binary tree.

Example 1:

1
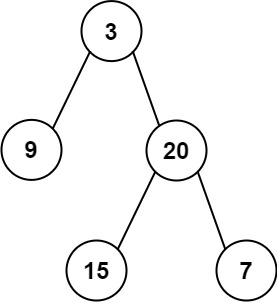
2
Input: inorder = [9,3,15,20,7], postorder = [9,15,7,20,3]
Output: [3,9,20,null,null,15,7]

Example 2:

1
2
Input: inorder = [-1], postorder = [-1]
Output: [-1]

Constraints:

  • 1 <= inorder.length <= 3000
  • postorder.length == inorder.length
  • -3000 <= inorder[i], postorder[i] <= 3000
  • inorder and postorder consist of unique values.
  • Each value of postorder also appears in inorder.
  • inorder is guaranteed to be the inorder traversal of the tree.
  • postorder is guaranteed to be the postorder traversal of the tree.

Hints/Notes

  • Draw the tree and find the pattern of root’s location

Solution

Language: C++

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    map<int, int> valToIndex;

    TreeNode* buildTree(vector<int>& inorder, vector<int>& postorder) {
        int size = inorder.size();
        for (int i = 0; i < size; i++) {
            valToIndex[inorder[i]] = i;
        }
        return build(inorder, 0, size - 1, postorder, 0, size - 1);
    }

    TreeNode* build(vector<int>& inorder, int inStart, int inEnd,
                    vector<int>& postorder, int postStart, int postEnd) {
        if (inStart > inEnd) {
            return nullptr;
        }

        int rootVal = postorder[postEnd];
        int rootIndex = valToIndex[rootVal];

        TreeNode* root = new TreeNode(rootVal);

        int leftSize = rootIndex - inStart;
        root->left = build(inorder, inStart, rootIndex - 1,
                           postorder, postStart, postStart + leftSize - 1);
        root->right = build(inorder, rootIndex + 1, inEnd,
                            postorder, postStart + leftSize, postEnd - 1);
        return root;
    }
};